Question The Curve Below Is The Graph Of (x^2 Y^2 1)^3 x^2 Y^3 = 0 (a) Sketch The Tangent Line To To Graph At The Point (1,1) (to) Find An Equation Of Line Which Is Tangent To The Graph At The Point (11) Protip Plug In (1,1) After Applying D/dx To Both Sides Of The Equation But Before Solving For Dy/dt Ex 21, 1 If (x/3 " 1, y –" 2/3) = (5/3 "," 1/3) , find the values of x and y (x/3 " 1, y –" 2/3) = (5/3 "," 1/3) Since the ordered pairs are equal, corresponding elements are equal Hence x/3 1 = 5/3 x/3 = 5/3 – 1 x/3 = 2/3 x = 2 y – 2/3 = 1/3Click here👆to get an answer to your question ️ If x,y,z are all different and if x & x^2 & 1 x^3 y & y^2 & 1 y^3 z & z^2 & 1 z^3 = 0 then 1 xyz =
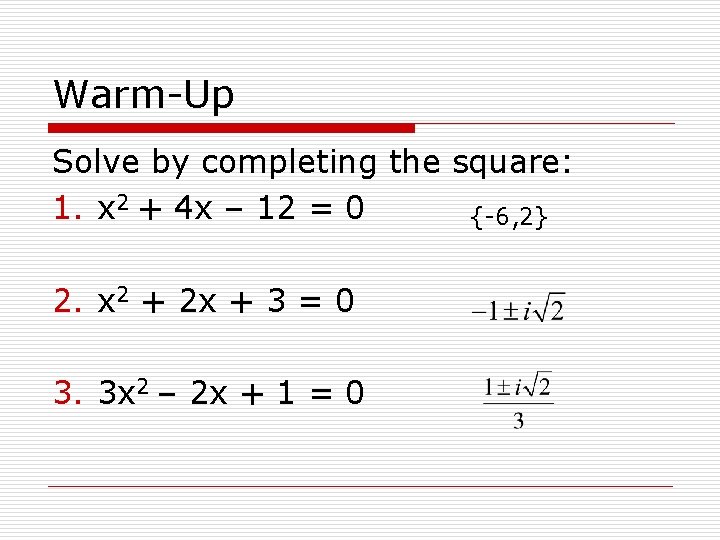
Warmup 1 Solve By Factoring 2 Write The